

The chain rule is very useful when you need to.
#CHAIN RULE CALCULUS HOW TO#
This section explains how to differentiate the function y sin(4x) using the chain rule. Join 23K views 3 years ago New Calculus Video Playlist This calculus video tutorial explains how to find derivatives using the chain rule. The chain rule can be generalised to multivariate functions, and represented by a tree diagram.
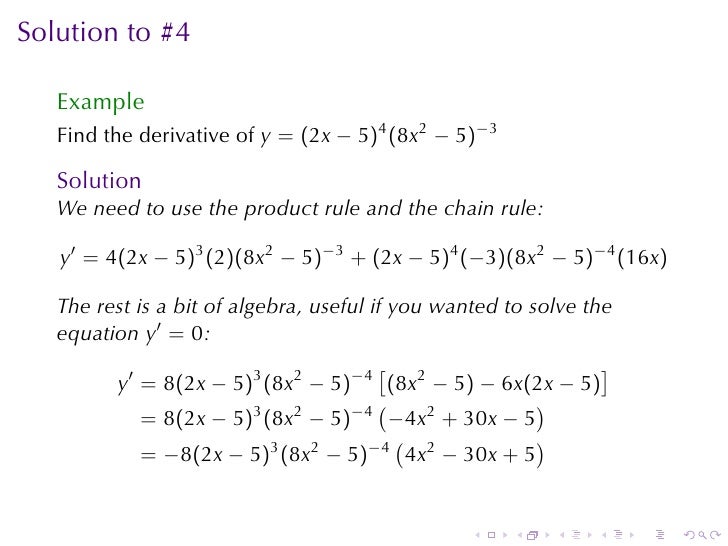
The answer is because we always use the chain rule.You must use the Chain rule to find the derivative of any function that is comprised of one function inside of another function. The chain rule in calculus is one way to simplify differentiation. The chain rule tells us how to obtain the derivative of a nested function y with respect to x like the following one. The chain rule allows us to find the derivative of a composite function. In particular, we will see that there are multiple variants to the chain rule here all depending on how many variables our function is dependent on and how each of those variables can, in turn, be written in terms of different variables. With the chain rule in hand we will be able to differentiate a much wider variety of functions. In calculus, the chain rule is a formula that expresses the derivative of the composition of two differentiable functions f and g in terms of the derivatives of. Share Cite Follow answered at 17:12 Cameron Williams 28.1k 4 54 103 Add a comment 7 Just to add on to the other answers: You ask why you need to use the chain rule. Chain Rule In this section we discuss one of the more useful and important differentiation formulas, The Chain Rule.
The product rule allows us to differentiate a function that includes the multiplication of two or more variables. You need to use chain rule because it is a composition of functions: f(x) ln(x) and g(x) 2x 1, so we see ln(2x 1) as f(g(x)). We derive each rule and demonstrate it with an example.

You ask why you need to use the chain rule. Instead of using the Chain Rule can't we use the rule applicable to logs: F (X)In (g (x)) F' (X) g' (x)/g (x) Therefore, using the example given: f (x) In (sin (x)) f' (x) cos (x)/sin (x) Is there anything wrong with using this method ( 4 votes) Ian Pulizzotto 2 years ago This method works. In the section we extend the idea of the chain rule to functions of several variables. Sharing is caringTweetIn this post, we are going to explain the product rule, the chain rule, and the quotient rule for calculating derivatives.


 0 kommentar(er)
0 kommentar(er)
